Volume of Basic Shapes

So today we looked at finding the volume of basic prisms. When I say basic prisms, what I mean are prisms that have a constant cross section. This means that the face you see on the shape is the same, no matter where you cut through the shape. For example;

The prism above has a pentagon as a face. If we were to cut a cross section at any point through this shape, it would have the same pentagon face no matter what. This works for a variety of shapes. For example; 

If a prism has this constant cross section, it is then known as a basic prism. 

To find the volume of these basic prisms we use the following formula; 

Volume = Area of Face x Height (or depth)

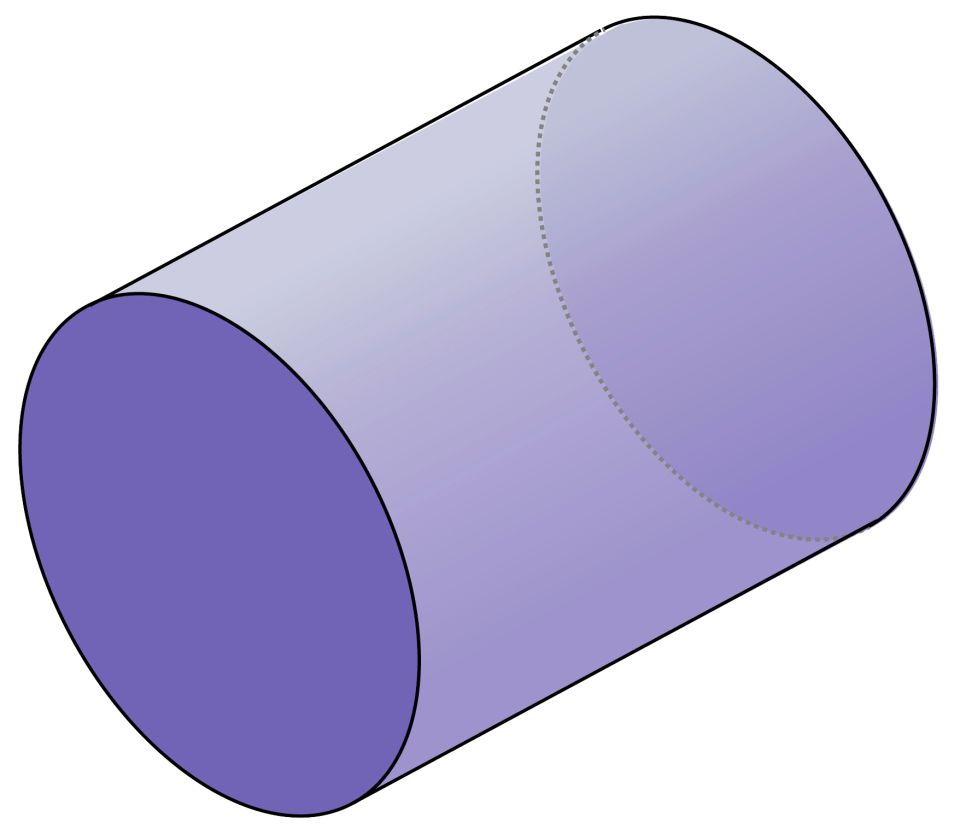
The face we find the area of is the face that has the constant cross section throughout the prism. For example, with the cylinder above we would find the area of the circular face, and then multiply it by the height or depth of the whole prism. 

Using this information, you should be able to make a start on the "Volume" questions on the first work sheet, which can be found in the "Work Sheets" tab. 

If you are still stuck on this topic, the following website is handy; 

http://www.mathsisfun.com/geometry/prisms.html

If you are still stuck, feel free to comment on this post, or email or chat to me in person.